Category Archives: Uncategorized
Open Letter to a Puppy: The Transformative Twos
My mayhems,
HAPPY JOINT SECOND BIRTHDAY!! What a joyous serendipity, to share the exact chronology of existence!
Oh, and Charlie: I’m pretty sure your birthdate is fraudulent. I don’t even know that you’re two yet.
See, your paperwork from the shelter lists your birthdate as 11/1/2020, but I suspect they figured out Jadie’s birthday and forged your documents to seal the adoption deal. I haven’t met a dog person yet who puts you north of 18 months.
But that’s the beauty of rescues: They fill whatever role they’re meant to play. And Chuck, yours is to be the 2-year-old; unpredictable, deliriously destructive and mouthy as hell. You still bark at the mail carrier every time, like you just saw Hitler in shorts. You eat my glasses. Hence and heretofore, your birthday is at midnight on Halloween, so we can still mark the occasion together while acknowledging the possibility of subterfuge.
And Jadie, of ruby lobes and Cali sunrise eyes, you are in full bloom. You have become the quiet(er), calm(er) sibling. You helped train Charlie on the dog door, taunting him with toys that you’d scamper inside. Now you both burst through the once-clear plastic flap like Starsky and Hutch on meth.
In fact, your first year together has been a bit like watching a 70’s cop show, where the patrol officers pretend to dislike each other. When we tool up for the park — leashes, music, water — you snap and snarl and growl at each other. You’ll grab each by the reins and drag the other to the door. ‘Why are you walking yourself? I’m not touching you. Does it bother you that you’re walking yourself? I’m not touching you.’
But then I open the hatchback, and you become synchronized swimmers, leaping and twisting and arcing leashward to the park, a place so sacred I have to say it in pig latin if it is spoken aloud. If I could rollerblade, we could Iditarod the 2-1/2 miles to the park, and we’d beat traffic (note to self: invent the rollerblade bobsled).
I’ll admit, I love the park, too, and not just for the fang and claw. The humans there, we’re all fractured in some way by a real world busted to bits. But we find grace in yours, where life is all windshield, no rearview mirror.
I try to imagine what that off-leash world is like. You lose your minds with unadulterated glee. Is it a Disneyland you vaguely recall, even though you were there just yesterday? A place where you know the rides by heart, but not what they do to yours?
Or do you remember everything, exactly, and it’s the memory that makes you that celebratory? I guess the answer doesn’t matter, but I wish you could see it.
Maybe you do. You both still have that verticality to your gallop, like you want to be airborne a moment longer, glimpse a moment extra, stretch a moment further. You both grin like hayseeds when you pant, so perhaps I’m anthropomorphizing a smile at the end of the day. But I could swear you dig Splash Mountain.
Anyway, happy second birthday! Who knows? To celebrate, we may go to the arkpay.
Pick an Atom, Any Atom
Evidentialism Factslap: There are more ways to arrange a deck of cards than there are atoms on Earth.
Think of your last card game – euchre, poker, Go Fish, whatever it was. Would you believe every time you gave the whole deck a proper shuffle, you were holding a sequence of cards which had never before existed in all of history? Consider how many card games must have taken place across the world since the beginning of humankind. No one has or likely ever will hold the exact same arrangement of 52 cards as you did during that game.
It seems unbelievable, but there are somewhere in the range of 8×1067 ways to sort a deck of cards. That’s an 8 followed by 67 zeros. To put that in perspective, even if someone could rearrange a deck of cards every second of the universe’s total existence, the universe would end before they would get even one billionth of the way to finding a repeat. This is the nature of probabilities with such great numbers. Though a long-time blackjack dealer might feel like they have shuffled thousands of cards in their lifetime, against a number this big, their rearrangements are irrelevant. There are simply too many ways to arrange 52 cards for any randomly organized set of cards to have repeated itself.
Don’t believe it? Consider how many ways you can order a deck of just four aces.
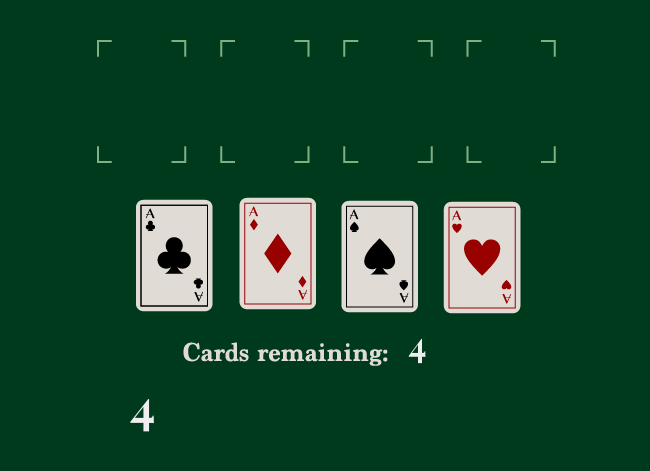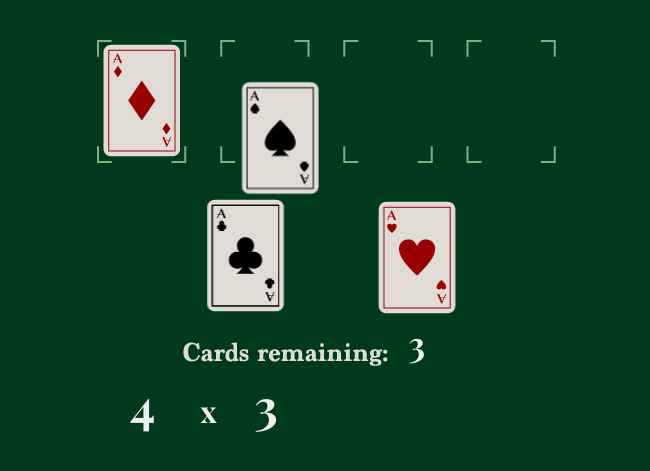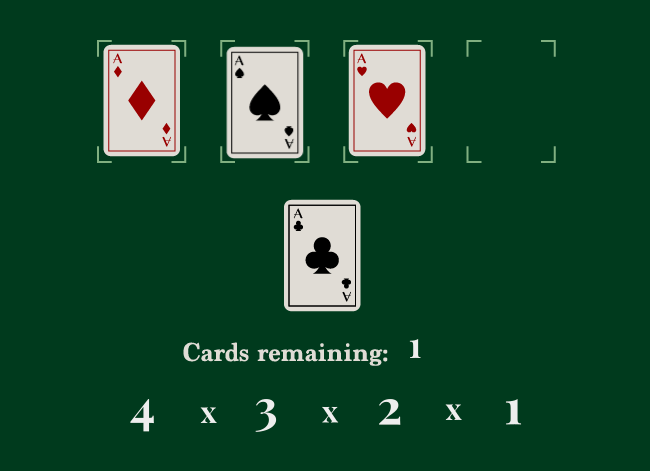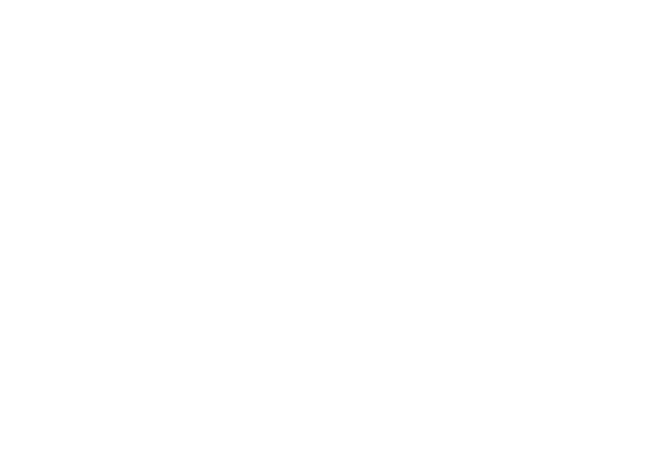
As you deal out the deck, each subsequent position in the row has one fewer card to select from. So the first spot has four options, the next spot has three, and so on until one card remains. This mathematical pattern can be used to calculate how many ways a set of things can be organized by multiplying these numbers together.
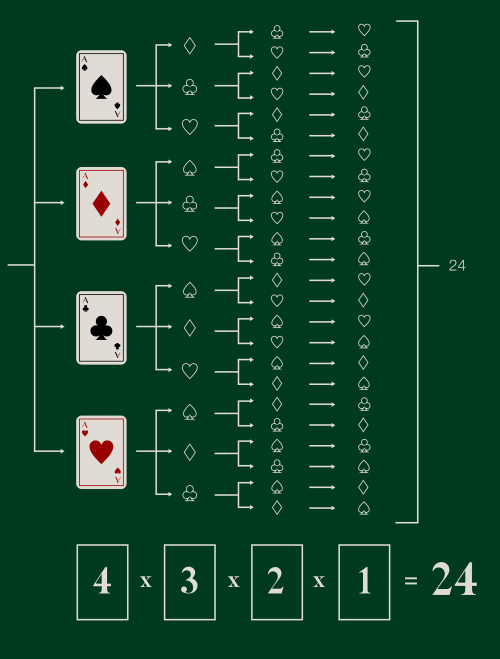
This literally exciting calculation is denoted by an exclamation mark and is called a factorial. As a rule, factorials multiply the number of things in a set by consecutively smaller numbers until 1. Since there are 4 cards in our mini-deck, there are 4 factorial or 4! numbers of ways it can be arranged, which equals 24. While this might not seem like a particularly large number, by the time you get to 52! (or 52x51x50 … ) you get a number with 68 total digits – an integer much larger than all the atoms estimated to be on Earth.
Now you might say, wait, 52 cards arranged in order from least to greatest cannot possibly be unique; you’ve probably even done it several times in your life playing Solitaire. And you’d be right. Card games tend to order decks in common ways, so you need to shuffle really well (about seven times using the “riffle” method) before you’re holding any deck which is truly one-of-a-kind





